Formula

Sources differ as to the exact formula for the Droop quota

• Henry Droop - English lawyer and mathematician - 1831-84
• quota = targeted minimum number of elected candidates

The formula used in the Republic of Ireland is usually written:

\left( \frac{Total Valid Poll }{\left( Seats+1 \right)} \right) + 1

The extra parentheses, while not strictly necessary from a mathematical standpoint, are often included in order to make the formula seem less ambiguous to non-mathematicians. If calculated out of sequence, an incorrect result would be arrived at, producing an incorrect quota. It is also crucially important when calculating to use the Total Valid Poll, which is arrived at by subtracting the spoiled and invalid votes from the total poll.

When calculated correctly, the Droop quota is the smallest number that guarantees that no more candidates can reach the quota than the number of seats available to be filled. From a strict mathematical point of view, the formula is best rendered:

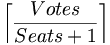
\left\lceil \frac{Votes}{Seats+1} \right\rceil

The brackets denote the operation of rounding up. This process is necessary because it not possible to have a fraction. Therefore if a mathematical  fraction results such as 7.3 or 7.7 the result is rounded up to 8. 

This gives the Droop quota the special property that it is the smallest integral (whole number) quota which guarantees that the number of candidates able to reach this quota cannot exceed the number of seats. In a single winner election, in which STV becomes the same as Instant Run-off Voting, the Droop quota becomes a simple integral majority quota–  that is, it will be equal to an absolute majority of votes.

While in theory every STV election should see the right number of candidates elected through reaching the quota, in practice many voters may only vote for a small proportion of the candidates on the ballot paper, such as only those candidates from one party, or even only one candidate. Those votes are known as 'NTs', or 'non transferable votes', and the effect of their removal from the total valid poll may be to reduce the total number of votes available to such an extent that the last candidate left in a race may not actually have enough votes to reach the quota. Nevertheless, in reality, as no other candidate may mathematically be able to overtake them as the candidate nearest to the quota, they may in such circumstances be deemed elected "without reaching the quota". The quota is in fact constructed to ensure that it is mathematically impossible for candidates to achieve the quota beyond the number of available seats.


Example

To see how the Droop quota works in an STV election imagine an election in which there are 2 seats to be filled and 3 candidates: Andrea, Carter and Brad. There are 102 voters. Two of these voters spoil their ballot papers. The remaining 100 voters vote as follows:

45 voters

  1. Andrea
  2. Carter

25 voters

  1. Carter

30 voters

  1. Brad

There are 102 voters but two spoil their papers so the Total Valid Poll is 100. There are 2 seats. Before rounding down the Droop quota is therefore:

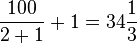
\frac{100}{2+1} + 1 = 34 \frac{1}{3}

Rounded down to the nearest integer the Droop quota is found to be 34. To begin the count the first preferences cast for each candidate are tallied and are as follows:

Andrea has more than 34 votes. She therefore has reached the quota and is declared elected. She has 11 votes more than the quota so these votes are transferred to Carter. The tallies therefore become:

Carter now has reached the quota so is declared elected. The winners of the election are therefore Andrea and Carter.